Foreword

   Resuming the discussion of dimensionality in terms of the topology of time, several dimension-like aspects of
time emerge.  Now the transitional zeroth dimension of
time - the time point - may be partially represented as the third dimension of space, for every event-point has some momentary solid state and configuration as its physical content.  The change of these 3-dimensional tableaux re- sults in occurrence.  Thus the first dimension of time may be likewise partially represented as a fourth dimension of space, whereby the juice enters the orange without pass- ing through the skin by the simple expedient of its having previously entered through the stem before the rind sealed itself off at the stem in ripening. The interoperation of negative and positive time axes explains such phenomena.
   The time-point is an instantaneous stage of an event - a 3-D tableau, the continuing occurrence of such point-events or changes forming a time line, curved or rotated into the second time dimension²⁸ by recurrence.

 28. This second temporal dimension is that of quantic or recurrent energy release. what we have before termed scope, the Y-axis of note 24; while the first temporal dimension refers to the X-axis.

        lviii

 This curvature is not simply closed (since there is never exact recurrence), but evoluted in a helicoidal skew-curve, constituting the third temporal dimension: development. Beyond this lies the envelope or carrier-wave of the de- velopment-helicoid, i.e. the fluctuating or pulsating pat- 
tern of possibility or ultimate, limiting cause which con-
trols development. 
      Finally and fifthly we have consummation, re-entry, or integration - the achieving of a unified relationship at every moment to all the past by exhibiting all past occur- 
rences as part of a single closed form and structure in this highest dimension of time, consummation, which is an ever re-creating ending, exhibiting the full implications of the beginning in now explicit form.  Such a continuous integration of all experienced phenomena is the primary function of consciousness through its various operational phases of perception, conception, memory, relation, emotion, volition and judgment.²⁹   Such integration thus leads to decision, creating another time-point; and the temporal dimensional cycle re-commences.  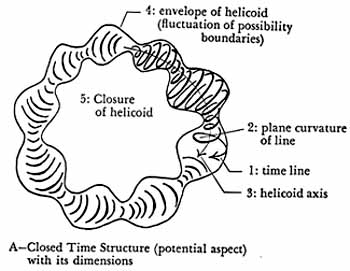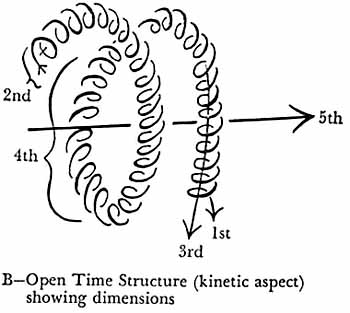There are two principal ways to display a 5-dimensional structure of time by means of 3-dimensional representations, as shown below.  Figure A represents a Closed-System model, referring intrinsically to the iniation-consummation of a particular development.  Figure B depicts an Open-System model, referring to the pattern of the functional structure itself, without special relation to a particular development from any epoch-event.  Summing up this reckoning, there are eight world dimensions in all, three of space and five of time.

29. The omission of this central fact of the integrating character of consciousness constitutes the cancer-like reductive fallacy of David Hume's thinking and of those who adopted it either in original or revamped form.

        lix

        lx

  30. Standing waves may be non-arbitrarily measured by closed curves or cycles. while the building up of increased amplitudes by resonance is measured by some evolving spiral. typically some functional form of ke^θ.  Similarly, interference or damping phenomena are measured by involving or whirlpool spirals.
  31. See note 24.

        lxii

ception of the phenomenon is accurate and clear enough, and the understanding of it full and deep enough, appar-
ent paradoxes will disappear, except in those regions where understanding is not adequate and problems still remain.  Paradoxes and dilemmas are to be preferred to even more arbitrarily reductive distortions, but they are by no means to be mistaken for final solutions or for the whole truth of their universes of discourse.  The reductive mind overlooks the return track of the railroad and then glories in the "insoluble paradox" that the trains must run both ways on the same track.  Until we cease desiring love affairs with paradoxes we shall never know the grace of truth, which follows only upon the unremittingly vigilant determination to omit nothing that exists from the framework of our conceptions, no matter how awkward that may be for our preconceptions.  For there is always a lot more that exists than what we are aware of at any given moment.  We can ill afford to forget that fact;  because if intellectual humility is thus lost, accuracy and logic are at the same moment jeopardized, and the probability of finding correct answers drastically reduced or nullified.
    Concluding the present discussion of potential energy and its direct bearing on chronotopology, we must realize that the usual statement, F = ma, does not define force, but represents only a meter-reading for force under certain conditions.  Thus the forces acting within a beam under stress are not taken into account by the ordinary appli- cation of that equation.  Again, a man holding up a roof from falling down will expend energy and become tired,

        lxiii

even though neither he nor the roof move.  His continu- ance of the standing waves of neuro-chemical energization of his muscle fibres causes him to become fatigued. Energy is thus expended even if only sub-observational standing waves are involved, making the phenomenon non-kinetic when macroscopically observed; and potential energy ap-
parently requires for its maintenance the expenditure of kinetic energy at a more microscopic level of observation.
Potential energy would thus be directly connected with increase of entropy - and hence with the movement of time - within a given region.³²
     A time factor as well as a distance factor must enter 
the picture, in combination with force, as a measure of energy. Now since by the usual definitions
                                   Ft = mv
we see that Force x Time has the dimensions of a momen-
tum, even, as in the case of the man holding up the roof, where no momentum is macroscopically observed.  Every momentum, mv, must by definition have an associated kinetic energy, ½ mv².  We can thus render the fact that potential energy is microscopically kinetic energy only by straining the ordinary conceptions, while chronotopologi-
cally such problems are removed by rendering the standing waves involved in explicit and calculable form.
     Considerable light is thrown at the same time on the nature of energy fields by these considerations;  as it is axiomatic, chronotopologically speaking, that all energy fields are maintained by and consist in standing waves, including their ultimate form as standing time waves.

    32.  See pages xiv-xv sup.

       lxiv

   Hence any given energy field possesses or is directly re- lated to at least one singularity or point-discontinuity showing up as a source or sink (cf. pp. li-lii).  That singu-
larity indicates the energic genesis of the standing waves.³³
We can now proceed another step to point out that not only fields, but resulting forms and structures, including biological ones, were ultimately created by standing waves of some kind, and are maintained by them - thus introduc- ing a natural order throughout a pervasive gamut of phe-
nomena.  That ultimate origin constitutes one of the rea- sons behind the extreme versatility of Fourier series in rep- resenting functions of all sorts, i.e. as a general measure.
      All natural morphological symmetry, bilateral or radial, is the result of some form of standing wave and may be mapped as a periodic function representable by a closed curve of some kind.³⁴  In general, all repetitive pattern whatsoever - of which symmetry is but one example - re-
sults from the action of some form of dynamic periodicity.³⁵
      Furthermore, the entire gamut of natural morphology

   33. To say that forces are due to "the metric of the field" is not only to lose sight of the energic nature of the field, but is moreover so much verbal smoke-screening until the ultimate energy sources maintaining and/or changing the metric are indicated, discussed, and brought to light.
     34. See also note 30.
     35. It is thus literally true, translating the basis of such a process from time waves to sound waves for the sake of more concrete imagery, that all natural forms were generated and are maintained by - music or song, in the most profound sense. Anciently we have the vak of the Hindu tantras, the enem of Sumerian cosmology, the heka of ancient Egypt, which, trans- planted and transformed by Greek thinkers, became the logos of Hellenic and Alexandrian thought from Pythagoras and Herakleitos to Plotinos and Philo ]udæus, and later, of the Christians.  It is interesting that practically every theology of the ancient world, including early Christianity, had hit upon this profound natural truth intuitively.  "And God said, Let there be light; and there was light."  The third word holds the sentence's power.
                                                                  lxv

appears to stem from the felicitous recombination of actu-
ally a few basic forms - archemorphs we may call them. These comparatively few archemorphs are found arranged and rearranged with each other, with appropriate thematic variations of form and size, much as the letters of the
alphabet in the various type styles and sizes of printing, - yielding the rich language of all the numberless natural 
forms of the world about us.  The forms of a perfect walnut meat removed from its shell, and of the human brain
removed from the skull, are examples of this subtle arche-
morphic variation.  A butterfly with outstretched wings
stands archemorphically between those two and a pair of
veined leaves on a stem, as well as the two lungs and tra- chea.  And the leaves are also the breathing organs of the tree.  Archemorphic interrelation is the key to the continu-
ity of all natural forms.  A vast field of mapping the wave
functions of archemorphs and their thematic variations
lies before us, from nuclei to nebulae.  Characteristic inte-
gers and certain common fractions, infinite series, and tran-
scendental numbers will become extremely important in
such investigations, as preliminary calculations have
proved.
       In conclusion, we previously briefly referred to the er-
rors attached to the generalizing of reductive formulas like
0 ± ki = 0 beyond their inherent limitations of applica-
tion.  Another specious example of the reductivist fallacy
arises from the ambiguity of the ordinary algebraic sign 
of equality.  Thus when we write
                                     e^πi=-1
we can by no means, as we have seen, equate the opera-

           lxvi

tional meaning of the two sides of the equation, but only
the end-results, and even then only as computed by one
necessarily limited aspect of those results; in this case, as
computed by the direction and magnitude of the coni-
cally rotating generating radius (see p. xlix above) at its
π-phase.  Again, though a + bi^-1 = a + bi³ = a - bi alge-
braically, they are severally three different operations or
paths leading to the same end-position.  The algebraic
equality sign refers to sameness of end-position, but does
not necessarily denote identity of the two or more processes equated. There is a further ambiguity as to units, and we write algebraically 1³ = 1² = 1, although geometrically it is not correct to state that a unit cube equals a unit square or a unit length.
   In physics mere algebraic equality is even less meaning-
ful than in geometry.  And chronotopological analysis,
depending as much upon the operational reasons for an
equality as on the mere algebraic equality, requires us to
look behind algebraic equality signs and in general to do
more than merely reductive expressions of either observa-
tion or conception permit.  This thought takes us back to
one of our initial distinctions: that between an existence
theorem and a reason theorem.  To uncover and exhibit
the fundamental, and not simply the tautological or for- 
mal reasons why a relation should be true requires mathe-
matical insight of a higher order than merely to demon-
strate that such a relation is true.  The latter type of
demonstration yields logical existence theorems, or simply
existence theorems; the former, reason theorems.

                                                                  lxvii

To Be Continued - Note: we could use a volunteer to continue this transcription.